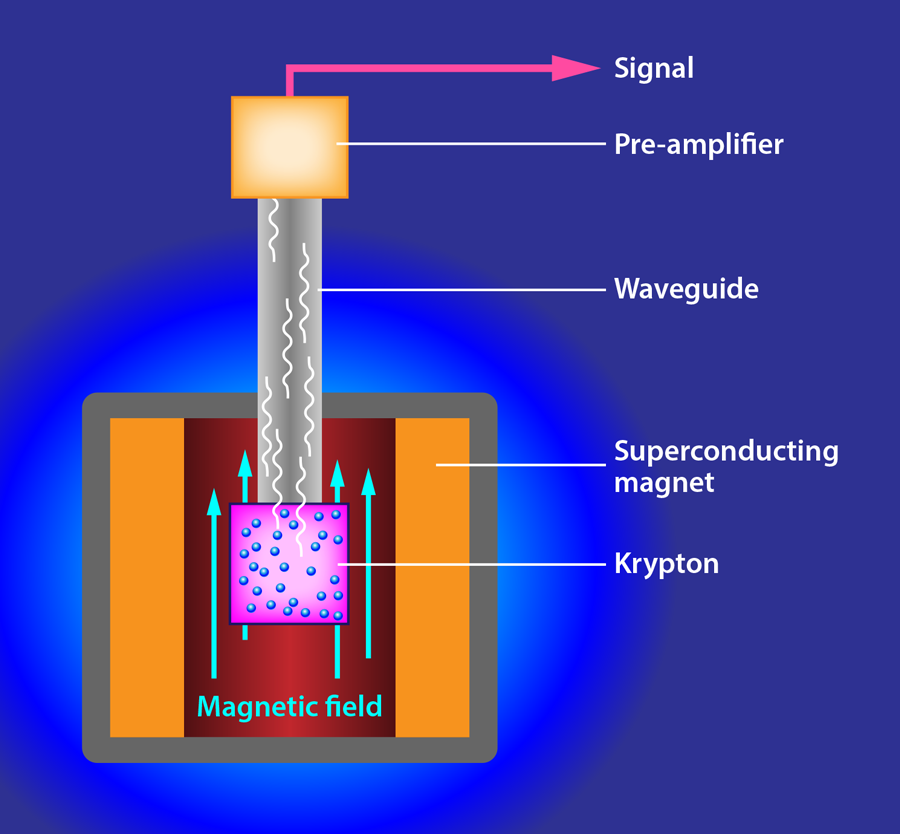
About Project 8

The goal of the Project 8 experiment is to measure the absolute neutrino mass using tritium beta decays. This technique, which involves precisely measuring the energies of the beta-decay electrons in the high-energy tail of the spectrum, has a history spanning more than 70 years. The approach to making that measurement taken by the Project 8 collaboration is to use a new method of electron spectroscopy, Cyclotron Radiation Emission Spectroscopy (CRES), which was first demonstrated by the collaboration in 2014.

In Project 8, we adopt the dictum of Arthur Schawlow: "Never measure anything but frequency!" In this case, we take advantage of the motion of charged particles in magnetic fields to make a measurement of their energies.

In a constant magnetic field, \(B\), the corresponding cyclotron motion of the electron occurs at a frequency that depends on the kinetic energy (\(K_e\)) of the charged particle:

\begin{equation} \label{eq:cyclotron} f_\gamma \equiv \frac{\omega_c}{2\pi \gamma} = \frac{1}{2\pi}\frac{e B}{m_e+K_e/c^2} \end{equation}

where \(e\ (m_e)\) is the electron charge (mass) and \(f_\gamma\) is the radiated cyclotron frequency. The baseline angular frequency \(\omega_c\) is \(1.759\times 10^{11}\) rad/s/T, which means at magnetic fields near 1 T, the emission is in the Ka microwave band. As the cyclotron frequency depends on the electron Lorentz factor, \(\gamma\), it is dependent on the kinetic energy of the electron. The corresponding radiation emitted by the electron motion is small enough not to significantly affect the electron's energy, yet still carries the information of the kinetic energy itself.

For a freely-radiating electron undergoing cyclotron motion, the total power \((P)\) radiated is given by the Larmor formula:

\begin{equation} P \propto \frac{2}{3} \frac{q^2 \omega_c^2 p_\perp^2}{m_e^2 c^3} \end{equation}

where \(p_\perp\) is is the relativistic transverse momentum of the trapped electron. For a 1 T magnetic field, the amount of power radiated by 30 keV electrons is approximately 1 fW.

In the limit of a perfectly uniform magnetic field, the achievable resolution on the total predicted by this technique depends the total observation time of the electron:

\begin{equation} \Delta \omega = \Gamma \equiv \frac{1}{\tau} \end{equation}

where \(\tau\) is the mean observation time of the electron.

The CRES technique offers distinct advantages over other spectroscopic techniques that are used in direct neutrino mass measurements:

1. Source = Detector -- There is no longer a need to separate the electrons from the radioactive source (a potential energy loss mechanism);
2. Frequency Measurement -- Frequency standards are some of the most accurate measurements we can make to date;
3. Full Spectrum Sampling -- We can in principle measure the entire beta decay spectrum at once, allowing us to leverage greater stability and statistics.

Phase I in our science program was to demonstrate the feasibility of CRES on single electrons emitted from a gaseous source (^83mKr). In Phase II we have made a measurement of the tritium spectrum using CRES. In Phase III we will demonstrate the ability to make CRES measurements in free space, as well as the ability to produce and trap atomic tritium. The final phase, Phase IV, will use atomic tritium to measure the neutrino mass with a sensitivity of approximately 40 meV.